
| Home > Gallery > My Models > Stellated DHI > "Dodecaplosion" | |
| Prev ("Hemi-Twister") | Next (Stella Octangula) |
"Dodecaplosion" |
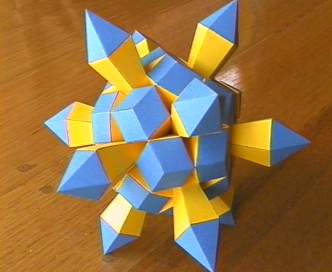
Yet another stunning stellation of the (small or great) dodecahemicosahedron. This model has crevices which reach all the way to the centre of the model.

|
Nets printed by Great Stella show tiny ticks along some edges (you might need to click on the image for a bigger version to see them). This shows where separate tabs are needed in cases where it would otherwise be impossible to tell. |

|
This is one of the spikes, looking like a torpedo, with the outer tabs glued inside and the inner tabs folded out as shown. Be careful when scoring these pieces! |

|
Here is the model partly finished. I started by using the tongue-in-groove method. This is a method for constructing models where some solid parts are only connected along an edge. On one part you glue the tabs folded out, as shown above, and on the other part the tabs fold in, but are not glued. Then you put glue on the out-folded tab and slot it into the other part. |

|
And this is the same partly finished model viewed from the other side, showing how all those crevices really do all meet right at the centre. |

|
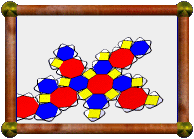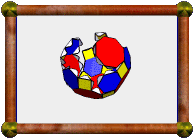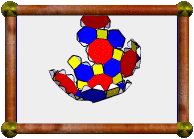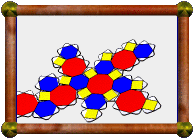
Getting there. |

|
Careful, the cat's got her eye on it. |

|
The tongue-in-groove method becomes too tricky before long, and you end up having to glue individual pieces. |

|
The view down a 5-fold rotational symmetry axes. |

|
The view down a 3-fold rotational symmetry axes. |

|
The view down a 2-fold rotational symmetry axes. |

|
This shows you how big the model is, in case you were wondering. |
| Home > Gallery > My Models > Stellated DHI > "Dodecaplosion" | |
| Prev ("Hemi-Twister") | Next (Stella Octangula) |