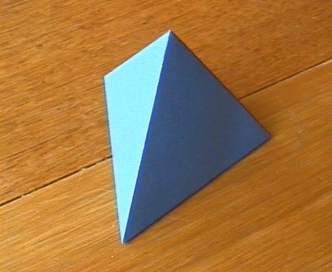
| Tetrahedron |
- Vertex description: 3.3.3
- Faces: 4
- Edges: 6
- Vertices: 4
- Dual: Tetrahedron (self-dual)
- Stellations:
- Fully supported: 1 (1 reflexible, 0 chiral)
- Miller's rules: 1 (1 reflexible, 0 chiral)
One of the five regular convex polyhedra known as the Platonic solids.
This model was made from a single connected net, printed on one sheet of A4
paper. Nets can be generated and printed at any size using any of
Small Stella,
Great Stella,
or
Stella4D,
even in the free demo versions.
|
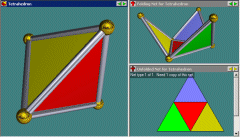
Here's a tetrahedron in Stella, using a 3-view layout featuring the
tetrahedron itself, the unfolded net, and a partially folded net.
See more screenshots here.
|

|
Multiple tetrahedra can be arranged in an intersecting manner to form
various compounds. The simplest and best known is the Stella
Octangula, consisting of 2 intersecting tetrahedra. This can be seen
as a compound of a tetrahedron with its dual, which happens to be
another tetrahedron. It is also a faceting of the
cube and a stellation of the
octahedron.
|

|
Here's another compound, consisting of 4 intersecting tetrahedra.
|

|
This compound consists of 5 intersecting tetrahedra. Since it has
rotational symmetry but no reflective symmetry, it comes in left and
right forms. It is a faceting of the
dodecahedron and a stellation of the
icosahedron.
|

|
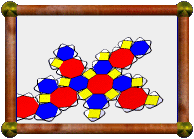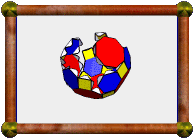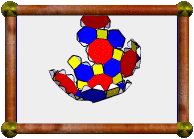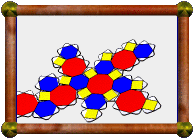
This compound contains 10 intersecting tetrahedra, and is a combination
of the two 5-tetrahedron compounds above. It is also a faceting of the
dodecahedron and a stellation of the
icosahedron.
|